组合总和

来源Leetcode第39题组合总和

给定一个无重复元素的数组 candidates 和一个目标数 target ，找出 candidates 中所有可以使数字和为 target 的组合。

candidates 中的数字可以无限制重复被选取。

说明：

• 所有数字（包括 target）都是正整数。
• 解集不能包含重复的组合。

示例 2:

输入: candidates = [2,3,5], target = 8,
所求解集为:
[
[2,2,2,2],
[2,3,3],
[3,5]
]

回溯

emm,这题想了想，要重复这一点属实把我难到了，一时没想出解决办法，就看了解答。

解答采用了回溯 + 剪枝的思路，其实回溯 + 剪枝在课设生成数独的时候做过了，然而已经完全忘了。
先上图

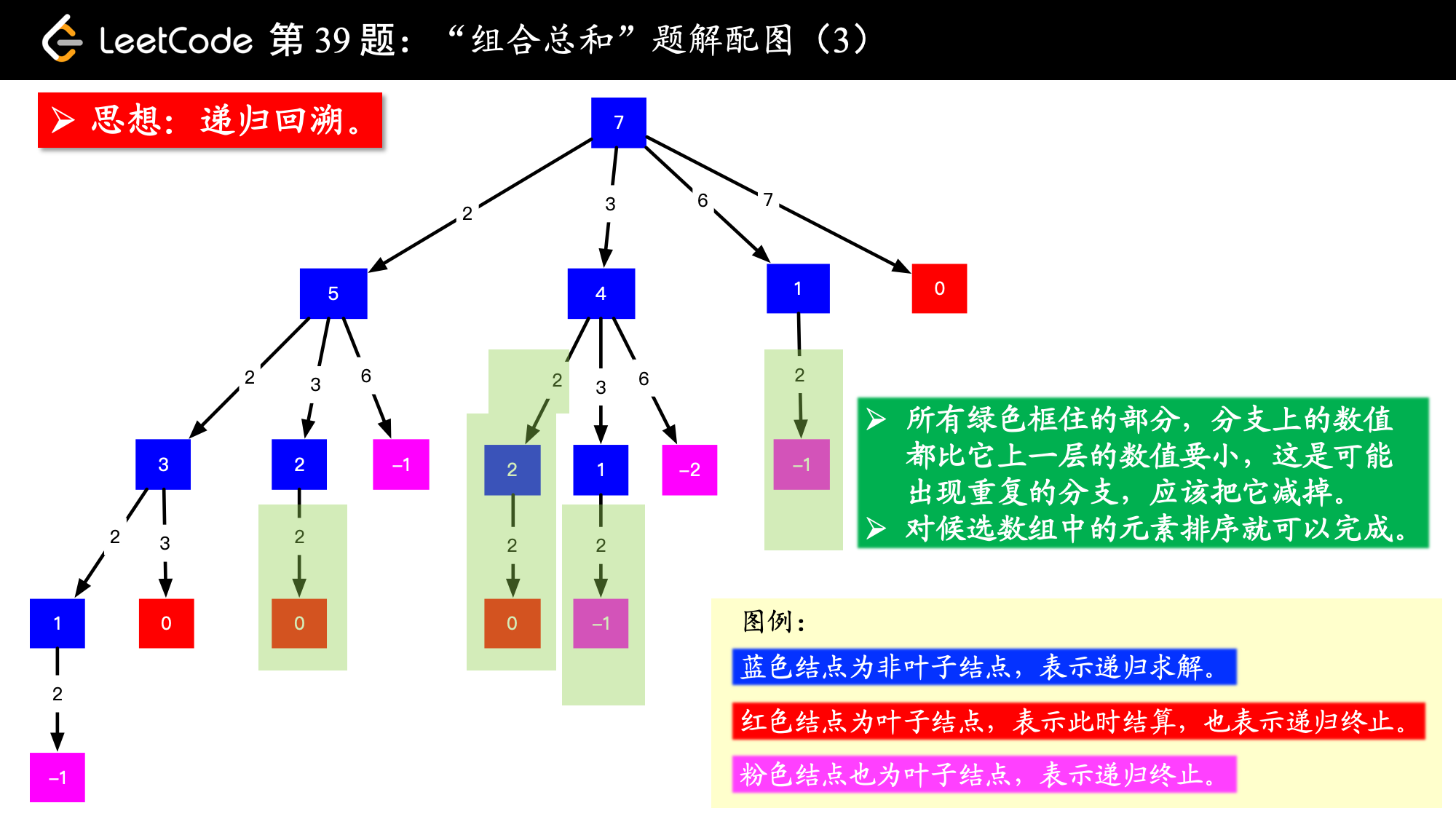
说明：

• 1.一个蓝色正方形表示的是 “尝试将这个数到数组 candidates 中找组合”，那么怎么找呢？挨个减掉那些数就可以了。

• 2.在减的过程中，会得到 00 和负数，也就是被我标红色和粉色的结点：

  • 得到 00 是我们喜欢的，从 00 这一点向根结点走的路径（很可能只走过一条边，也算一个路径），就是一个组合，在这一点要做一次结算（把根结点到 00 所经过的路径，加入结果集）。

  • 得到负数就说明这条路走不通，没有必要再走下去了。

总结一下：在减的过程中，得到 00 或者负数，就没有必要再走下去，所以这两种情况就分别表示成为叶子结点。此时递归结束，然后要发生回溯。

代码如下：

public class Solution {

    private List<List<Integer>> res = new ArrayList<>();
    private int[] candidates;
    private int len;

    private void findCombinationSum(int residue, int start, Stack<Integer> pre) {
		if(residue < 0)
			return;
        if (residue == 0) {
            // Java 中可变对象是引用传递，因此需要将当前 path 里的值拷贝出来
            res.add(new ArrayList<>(pre));
            return;
        }
        // 优化添加的代码2：在循环的时候做判断，尽量避免系统栈的深度
        // residue - candidates[i] 表示下一轮的剩余，如果下一轮的剩余都小于 0 ，就没有必要进行后面的循环了
        // 这一点基于原始数组是排序数组的前提，因为如果计算后面的剩余，只会越来越小
        for (int i = start; i < len && residue - candidates[i] >= 0; i++) {
            pre.add(candidates[i]);
            // [关键]因为元素可以重复使用，这里递归传递下去的是 i 而不是 i + 1
            findCombinationSum(residue - candidates[i], i, pre);
            pre.pop();
        }
    }

    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        int len = candidates.length;
        if (len == 0) {
            return res;
        }
        Arrays.sort(candidates);
        this.len = len;
        this.candidates = candidates;
        findCombinationSum(target, 0, new Stack<>());
        return res;
    }
}

栈

这是没有用栈的：

class Solution {
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        List<List<Integer>> res = new ArrayList<>();
        Arrays.sort(candidates);
        backtrack(candidates, target, res, 0, new ArrayList<Integer>());
        return res;
    }

    private void backtrack(int[] candidates, int target, List<List<Integer>> res, int i, ArrayList<Integer> tmp_list) {
        if (target < 0) return;
        if (target == 0) {
            res.add(new ArrayList<>(tmp_list));
            return;
        }
        for (int start = i; start < candidates.length; start++) {
            if (target < candidates[start]) break;
			//从第0个元素开始进行递归
            tmp_list.add(candidates[start]);
			//采用减法进行回溯
            backtrack(candidates, target - candidates[start], res, start, tmp_list);
			//删去最后一个新添加的元素，然后进行下一层的回溯
            tmp_list.remove(tmp_list.size() - 1);
        }
    }
}